Knaster–Kuratowski–Mazurkiewicz lemma
The Knaster–Kuratowski–Mazurkiewicz lemma is a basic result in mathematical fixed-point theory published in 1929 by Knaster, Kuratowski and Mazurkiewicz in:
B. Knaster, C. Kuratowski, and S. Mazurkiewicz, Ein Beweis des Fixpunktsatzes für n-dimensionale Simplexe, Fund. Math. 14 (1929) 132–137.
The KKM lemma can be proved from Sperner's lemma and can be used to prove the Brouwer fixed point theorem.
KKM Lemma. Suppose that a simplex 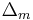 is covered by the closed sets
is covered by the closed sets 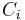 for
for  and that for all
and that for all 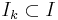 the face of S that is spanned by
the face of S that is spanned by 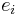 for
for  is covered by
is covered by 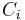 for
for  then all the
then all the 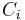 have a common intersection point.
have a common intersection point.
The two-dimensional case may serve as an illustration. In this case the simplex 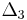 is a triangle, whose vertexes we can label 1, 2 and 3. We are given three closed sets
is a triangle, whose vertexes we can label 1, 2 and 3. We are given three closed sets  which collectively cover the triangle; also we are told that
which collectively cover the triangle; also we are told that 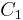 covers vertex 1,
covers vertex 1, 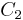 covers vertex 2,
covers vertex 2, 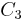 covers vertex 3, and that the edge 12 (from vertex 1 to vertex 2) is covered by the sets
covers vertex 3, and that the edge 12 (from vertex 1 to vertex 2) is covered by the sets 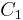 and
and 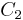 , the edge 23 is covered by the sets
, the edge 23 is covered by the sets 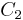 and
and 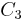 , the edge 31 is covered by the sets
, the edge 31 is covered by the sets 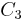 and
and 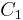 . The KKM lemma states that the sets
. The KKM lemma states that the sets  have at least one point in common.
have at least one point in common.
References
- See the proof of KKM Lemma in Planet Math.